| 3.8.9 define-compiler-macro | Macro |
- Syntax:
-
define-compiler-macro
name lambda-list [[{declaration}* $|$ documentation]] {form}*
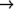 name
name
- Arguments and Values:
-
name - a function name.
lambda-list - a macro lambda list.
declaration - a declare expression; not evaluated.
documentation - a string; not evaluated.
form - a form.
- Description:
-
This is the normal mechanism for defining a compiler macro function. Its manner of definition is the same as for defmacro; the only differences are:
- The name can be a function name naming
any function or macro.
- The expander function is installed as a compiler macro function
for the name, rather than as a macro function.
- The &whole argument is bound to the form argument that
is passed to the compiler macro function. The remaining lambda-list
parameters are specified as if this form contained the function name in the
car and the actual arguments in the cdr, but if the car
of the actual form is the symbol funcall, then the destructuring of
the arguments is actually performed using its cddr instead.
-
Documentation is attached as a documentation string
to name (as kind compiler-macro)
and to the compiler macro function.
- Unlike an ordinary macro, a compiler macro can decline to provide an expansion merely by returning a form that is the same as the original (which can be obtained by using &whole).
- The name can be a function name naming
any function or macro.
- Examples:
-
(defun square (x) (expt x 2))
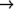 SQUARE
(define-compiler-macro square (&whole form arg)
(if (atom arg)
`(expt ,arg 2)
(case (car arg)
(square (if (= (length arg) 2)
`(expt ,(nth 1 arg) 4)
form))
(expt (if (= (length arg) 3)
(if (numberp (nth 2 arg))
`(expt ,(nth 1 arg) ,(* 2 (nth 2 arg)))
`(expt ,(nth 1 arg) (* 2 ,(nth 2 arg))))
form))
(otherwise `(expt ,arg 2)))))
SQUARE
(define-compiler-macro square (&whole form arg)
(if (atom arg)
`(expt ,arg 2)
(case (car arg)
(square (if (= (length arg) 2)
`(expt ,(nth 1 arg) 4)
form))
(expt (if (= (length arg) 3)
(if (numberp (nth 2 arg))
`(expt ,(nth 1 arg) ,(* 2 (nth 2 arg)))
`(expt ,(nth 1 arg) (* 2 ,(nth 2 arg))))
form))
(otherwise `(expt ,arg 2))))) 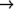 SQUARE
(square (square 3))
SQUARE
(square (square 3)) 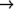 81
(macroexpand '(square x))
81
(macroexpand '(square x)) 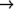 (SQUARE X), false
(funcall (compiler-macro-function 'square) '(square x) nil)
(SQUARE X), false
(funcall (compiler-macro-function 'square) '(square x) nil)
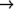 (EXPT X 2)
(funcall (compiler-macro-function 'square) '(square (square x)) nil)
(EXPT X 2)
(funcall (compiler-macro-function 'square) '(square (square x)) nil)
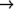 (EXPT X 4)
(funcall (compiler-macro-function 'square) '(funcall #'square x) nil)
(EXPT X 4)
(funcall (compiler-macro-function 'square) '(funcall #'square x) nil)
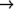 (EXPT X 2)
(defun distance-positional (x1 y1 x2 y2)
(sqrt (+ (expt (- x2 x1) 2) (expt (- y2 y1) 2))))
(EXPT X 2)
(defun distance-positional (x1 y1 x2 y2)
(sqrt (+ (expt (- x2 x1) 2) (expt (- y2 y1) 2))))
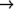 DISTANCE-POSITIONAL
(defun distance (&key (x1 0) (y1 0) (x2 x1) (y2 y1))
(distance-positional x1 y1 x2 y2))
DISTANCE-POSITIONAL
(defun distance (&key (x1 0) (y1 0) (x2 x1) (y2 y1))
(distance-positional x1 y1 x2 y2))
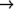 DISTANCE
(define-compiler-macro distance (&whole form
&rest key-value-pairs
&key (x1 0 x1-p)
(y1 0 y1-p)
(x2 x1 x2-p)
(y2 y1 y2-p)
&allow-other-keys
&environment env)
(flet ((key (n) (nth (* n 2) key-value-pairs))
(arg (n) (nth (1+ (* n 2)) key-value-pairs))
(simplep (x)
(let ((expanded-x (macroexpand x env)))
(or (constantp expanded-x env)
(symbolp expanded-x)))))
(let ((n (/ (length key-value-pairs) 2)))
(multiple-value-bind (x1s y1s x2s y2s others)
(loop for (key) on key-value-pairs by #'cddr
count (eq key ':x1) into x1s
count (eq key ':y1) into y1s
count (eq key ':x2) into x2s
count (eq key ':y1) into y2s
count (not (member key '(:x1 :x2 :y1 :y2)))
into others
finally (return (values x1s y1s x2s y2s others)))
(cond ((and (= n 4)
(eq (key 0) :x1)
(eq (key 1) :y1)
(eq (key 2) :x2)
(eq (key 3) :y2))
`(distance-positional ,x1 ,y1 ,x2 ,y2))
((and (if x1-p (and (= x1s 1) (simplep x1)) t)
(if y1-p (and (= y1s 1) (simplep y1)) t)
(if x2-p (and (= x2s 1) (simplep x2)) t)
(if y2-p (and (= y2s 1) (simplep y2)) t)
(zerop others))
`(distance-positional ,x1 ,y1 ,x2 ,y2))
((and (< x1s 2) (< y1s 2) (< x2s 2) (< y2s 2)
(zerop others))
(let ((temps (loop repeat n collect (gensym))))
`(let ,(loop for i below n
collect (list (nth i temps) (arg i)))
(distance
,@(loop for i below n
append (list (key i) (nth i temps)))))))
(t form))))))
DISTANCE
(define-compiler-macro distance (&whole form
&rest key-value-pairs
&key (x1 0 x1-p)
(y1 0 y1-p)
(x2 x1 x2-p)
(y2 y1 y2-p)
&allow-other-keys
&environment env)
(flet ((key (n) (nth (* n 2) key-value-pairs))
(arg (n) (nth (1+ (* n 2)) key-value-pairs))
(simplep (x)
(let ((expanded-x (macroexpand x env)))
(or (constantp expanded-x env)
(symbolp expanded-x)))))
(let ((n (/ (length key-value-pairs) 2)))
(multiple-value-bind (x1s y1s x2s y2s others)
(loop for (key) on key-value-pairs by #'cddr
count (eq key ':x1) into x1s
count (eq key ':y1) into y1s
count (eq key ':x2) into x2s
count (eq key ':y1) into y2s
count (not (member key '(:x1 :x2 :y1 :y2)))
into others
finally (return (values x1s y1s x2s y2s others)))
(cond ((and (= n 4)
(eq (key 0) :x1)
(eq (key 1) :y1)
(eq (key 2) :x2)
(eq (key 3) :y2))
`(distance-positional ,x1 ,y1 ,x2 ,y2))
((and (if x1-p (and (= x1s 1) (simplep x1)) t)
(if y1-p (and (= y1s 1) (simplep y1)) t)
(if x2-p (and (= x2s 1) (simplep x2)) t)
(if y2-p (and (= y2s 1) (simplep y2)) t)
(zerop others))
`(distance-positional ,x1 ,y1 ,x2 ,y2))
((and (< x1s 2) (< y1s 2) (< x2s 2) (< y2s 2)
(zerop others))
(let ((temps (loop repeat n collect (gensym))))
`(let ,(loop for i below n
collect (list (nth i temps) (arg i)))
(distance
,@(loop for i below n
append (list (key i) (nth i temps)))))))
(t form))))))
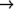 DISTANCE
(dolist (form
'((distance :x1 (setq x 7) :x2 (decf x) :y1 (decf x) :y2 (decf x))
(distance :x1 (setq x 7) :y1 (decf x) :x2 (decf x) :y2 (decf x))
(distance :x1 (setq x 7) :y1 (incf x))
(distance :x1 (setq x 7) :y1 (incf x) :x1 (incf x))
(distance :x1 a1 :y1 b1 :x2 a2 :y2 b2)
(distance :x1 a1 :x2 a2 :y1 b1 :y2 b2)
(distance :x1 a1 :y1 b1 :z1 c1 :x2 a2 :y2 b2 :z2 c2)))
(print (funcall (compiler-macro-function 'distance) form nil)))
DISTANCE
(dolist (form
'((distance :x1 (setq x 7) :x2 (decf x) :y1 (decf x) :y2 (decf x))
(distance :x1 (setq x 7) :y1 (decf x) :x2 (decf x) :y2 (decf x))
(distance :x1 (setq x 7) :y1 (incf x))
(distance :x1 (setq x 7) :y1 (incf x) :x1 (incf x))
(distance :x1 a1 :y1 b1 :x2 a2 :y2 b2)
(distance :x1 a1 :x2 a2 :y1 b1 :y2 b2)
(distance :x1 a1 :y1 b1 :z1 c1 :x2 a2 :y2 b2 :z2 c2)))
(print (funcall (compiler-macro-function 'distance) form nil)))
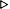 (LET ((#:G6558 (SETQ X 7))
(LET ((#:G6558 (SETQ X 7))
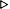 (#:G6559 (DECF X))
(#:G6559 (DECF X))
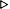 (#:G6560 (DECF X))
(#:G6560 (DECF X))
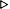 (#:G6561 (DECF X)))
(#:G6561 (DECF X)))
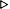 (DISTANCE :X1 #:G6558 :X2 #:G6559 :Y1 #:G6560 :Y2 #:G6561))
(DISTANCE :X1 #:G6558 :X2 #:G6559 :Y1 #:G6560 :Y2 #:G6561))
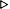 (DISTANCE-POSITIONAL (SETQ X 7) (DECF X) (DECF X) (DECF X))
(DISTANCE-POSITIONAL (SETQ X 7) (DECF X) (DECF X) (DECF X))
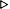 (LET ((#:G6567 (SETQ X 7))
(LET ((#:G6567 (SETQ X 7))
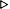 (#:G6568 (INCF X)))
(#:G6568 (INCF X)))
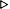 (DISTANCE :X1 #:G6567 :Y1 #:G6568))
(DISTANCE :X1 #:G6567 :Y1 #:G6568))
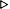 (DISTANCE :X1 (SETQ X 7) :Y1 (INCF X) :X1 (INCF X))
(DISTANCE :X1 (SETQ X 7) :Y1 (INCF X) :X1 (INCF X))
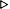 (DISTANCE-POSITIONAL A1 B1 A2 B2)
(DISTANCE-POSITIONAL A1 B1 A2 B2)
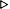 (DISTANCE-POSITIONAL A1 B1 A2 B2)
(DISTANCE-POSITIONAL A1 B1 A2 B2)
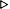 (DISTANCE :X1 A1 :Y1 B1 :Z1 C1 :X2 A2 :Y2 B2 :Z2 C2)
(DISTANCE :X1 A1 :Y1 B1 :Z1 C1 :X2 A2 :Y2 B2 :Z2 C2)
 NIL
NIL
- See Also:
-
compiler-macro-function,
defmacro,
documentation,
Section 3.4.11 Syntactic Interaction of Documentation Strings and Declarations
- Notes:
-
The consequences of writing a compiler macro definition for a function
in the common-lisp package are undefined; it is quite possible that in some
implementations such an attempt would override an equivalent or equally
important definition. In general, it is recommended that a programmer only
write compiler macro definitions for functions he or she personally
maintains--writing a compiler macro definition for a function maintained
elsewhere is normally considered a violation of traditional rules of modularity
and data abstraction.
- Allegro CL Implementation Details:
- None.